
What are factors, factor pairs, prime factorization, and how to find them? In this blog post, you will learn everything about factors of 24. Once you know how to find factors of numbers, you can quickly solve questions and practice finding factors of all numbers with your private math tutor. Let’s start with the basic concept of the factor with quick tips.
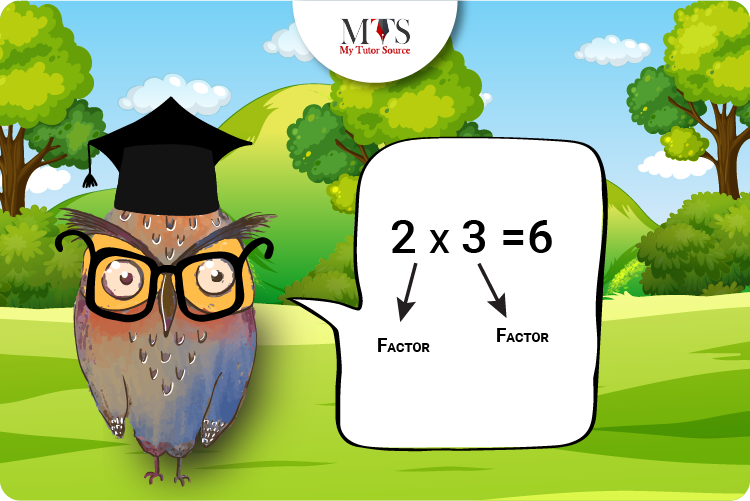
Multiplying two integers gives a product. The integers we multiply are known as the factors of the product. 1 and the number itself always counts as factors.
For example
4 x 2 = 8
Here, 4 and 2 are the factors of 8.
Moreover, 1 and 8 are also the factors of 8.
Keeping the definition of factors in mind, the factors of 24 are the numbers (positive or negative whole numbers) that are evenly divisible by the number 24.
Take numbers one by one and divide them by 24. If the remainder is 0 (zero), that means the divisor is the factor of 24.
Let’s get started:
Hence, the factors of 24 are 1, 2, 3, 4, 6, 8, 12, 24

Pairs of a number multiplied together to give the original number are known as factor pairs. In other words, combinations of two factors that give the product of actual numbers are called factor pairs. There are both negative and positive factors of a number.
Following are the combination of two factors or factor pairs, both positive and possible negative factor pairs of 24, that you must know about:
| Positive Factor Pairs | Negative Factor Pairs |
| 1 x 24 = 24 | -1 x -24 = 24 |
| 2 x 12 = 24 | -2 x -12 = 24 |
| 3 x 8 = 24 | -3 x -8 = 24 |
| 4 x 6 = 24 | -4 x -6 = 24 |
| 8 x 3 = 24 | -8 x -3 = 24 |
| 12 x 2 = 24 | -12 x -2 = 24 |
| 24 x 1 = 24 | -24 x -1 = 24 |
Thus, the pair factors are represented as
Finding which prime numbers multiply together to give or make the original number is known as prime factorization. Sometimes numbers need to repeat to write the prime factors of a number. Also, every number is a prime number in prime factorization. You can find the prime factorization of both composite numbers and prime numbers.
The prime factorization of 24 is 2 x 2 x 2 x 3 or 23 x 3
Here, 2 and 3 are the prime numbers even when 24 itself is a composite number.
24 is a composite number, and here are a few steps to find its prime factors:
Note

Question:
Jack has 24 chocolate cookies in his lunch box. He has to distribute the cookies among his 8 friends. How can he do it?
Solution:
Number of cookies Jack has = 24
Number of his friends = 8
Each friend will get = 24/8 = 3
Hence, each of Jack’s friends will get 3 cookies.

Question:
Find the sum of factors of 24?
Solution:
As the factors of 24 are 1, 2, 3, 4, 6, 8, 12, and 24.
So,
Sum of its factors= 1+2+3+4+6+8+12+24 = 60
Thus, 60 is the sum of factors of 24.

Question:
Find the common factors of 24 and 12?
Solution:
Factors of 24: 1, 2, 3, 4, 6, 8, 12, 24
Factors of 12: 1, 2, 3, 4, 6, 12
Thus, 1, 2, 3, 4, 6, and 12 are the common factors in numbers 24 and 12.

To find a factor of any number, simply find out what numbers should be multiplied together to get that number.