
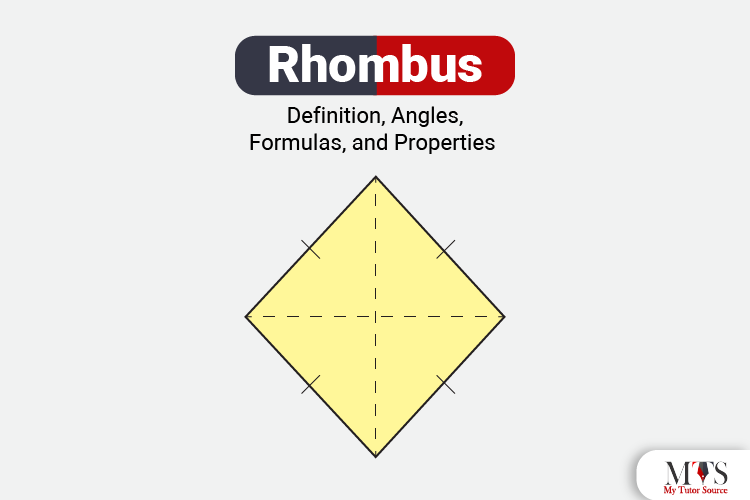
Are you finding difficulties in understanding the concept of a Rhombus? If yes, then you landed at the right place! The rhombus is a type of quadrilateral and usually has a diamond shape. It is one of the most commonly used Quadrilateral shapes in Mathematics and Geometry. Moreover, all the diagonals in a rhombus intersect with each other at a 90 degrees angle.
In this post, we are going to discuss and explain the definition, angles, formulas, and properties of a rhombus. Trust me; you will feel like you are having the easiest lesson of your life from a professional Math tutor while reading this post! But before going into the details of a rhombus, let us take a quick look at what is a quadrilateral!
What is a Quadrilateral?
A quadrilateral is a closed shape that has four angles enclosed with four verticals and four sides. Moreover, when you sum up all the interior angles of the quadrilateral, the answer would be equal to 360 degrees. There are further 6 types of quadrilateral:
A rhombus is defined as a type of quadrilateral, and a special type of parallelogram as well. All the opposite sides in a rhombus are parallel, and all the opposite angles are equal. Also, all four sides in a rhombus have equal and same length. Additionally, all the diagonals of a rhombus bisect each other at right angles.
Moreover, a Rhombus has three additional names; since a rhombus has a diamond shape, it is also called a ‘rhombus diamond’, Lozenge, or simply a ‘diamond’. In plural form, it is called rhombuses or rhombi.
All the sides of a rhombus are equal, right? Well, so does a square. Not only this, but all the diagonals bisect the opposite angles of the square. Also, a square has four right angles as well. Hence, a square can definitely be a type of Rhombus.
We hope that you already know a rhombus has four interior angles, right? Now let us go through some significant facts about Rhombus angles:
There are two basic Rhombus formulas to find out these two things:
Here is how you can find these both things:
The region that a rhombus covers in a two-dimensional plane is known as the area of a rhombus. Here is the formula to find it:
Area of a Rhombus = A = (d1 x d2)/2 square units
In this formula, d1 and d2 are known as the diagonals.
The total length of the boundaries of a Rhombus shape is its perimeter. In simpler words, the sum of all the four sides of a rhombus is known as its perimeter. Here is the formula to find the perimeter:
The perimeter of a Rhombus = P = 4a units
In this formula, ‘a’ is the side.
Now that we have gone through the definition, angles, and formulas, let’s move to the properties of a Rhombus! All these following properties are extremely important and you should know them by heart to fully absorb the concept of a Rhombus. Read on!
Here are a few solved problems and examples related to the area and the perimeter of a Rhombus to help you have a better understanding!
Question no. 1
The diagonal lengths of a Rhombus (d1 and d2) are 7 cm and 15 cm. What is the area of this Rhombus?
Solution:
d1 = 7 cm
d2 = 15 cm
Now, we apply the formula:
A = (d1 x d2)/2 square units
A = ( 7 x 15)/2
A = 105/2
A = 52.5 cm2
Question no. 2
If the area of a Rhombus is 90 cm2, and the length of its longest diagonal is 15 cm. What is the diagonal of this Rhombus?
Solution:
Here, area of Rhombus = 90 cm2 and supposedly d1 = 15 cm.
Now, we apply the formula:
A = (d1 x d2)/2 square units
90 = (15 x d2)/2
121 = 7.5 x d2
or 7.5 = d2
Thus, the diagonal of this Rhombus is 7.5.
Question no. 3
If all the sides of a Rhombus are 8 cm, then what will be its perimeter?
Solution:
Side of the Rhombus = 8 cm
Now, we know that all sides are equal. Hence, we apply the formula:
Perimeter = 4 x side
P = 4 x 8
P = 32 cm
Hence, the perimeter of the Rhombus is 32 cm.
Not a type, but yes, a square can be a rhombus.
No, a Rhombus can never have 4 right angles.
No, only the opposite angles of a rhombus are equal.
Now that you have gone through the entire post, we are sure your concepts about the definition, angles, formulas, and properties of a Rhombus are cleared. For more easy and detailed lessons, keep following and checking our blog or book one of our professional math tutors. You will surely find the answers to everything!